This post is a follow-up to Sarah Stephens’ guest post of a week ago, in which she described a lesson using embodied cognition to help students make sense of the interior angle sum theorem for triangles, not just as an abstract concept, but as a property grounded in their concrete physical experiences.

The day before publishing Sarah’s post, my wife Nan and I were visiting our grandchildren Sebastian and Darien, so I invited them to help me figure something out about the angles of a triangle. They agreed, and we tied a 20-foot-long string into a loop. Each of us held a spot on the rope, and we separated to pull the string taught, making a triangle with nice straight sides. We adjusted our holds a bit so that the sides were all of different lengths, and we checked out the angles by looking at each other. First Darien looked at Sebastian and then at me, and thought about how much of a turn he had made, and then Sebastian and I each took our turn doing the same thing. We talked about who had to turn the most, and who had to turn the least, as we changed the direction we were looking. We then did the turns again, this time using our arms. (Sebastian pointed his arm at me, then turned it to point at Darien, and Darien and I then did likewise.)

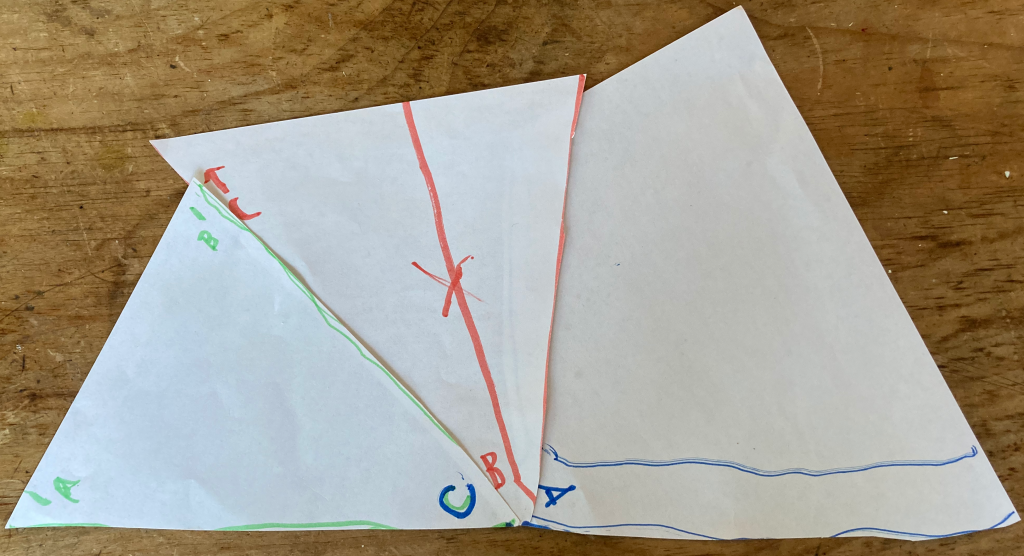
We talked about how we could record these angles on paper, and decided (with a hint or two from me) to lower our triangle down onto the floor, putting a piece of paper underneath each vertex. We made sure the triangle was tight, and then traced along the string to show the starting and ending directions for the turns each of us had made. Finally we cut the paper along the lines so that the only part left of each paper was the part inside the triangle, and we put the vertex points of the resulting pieces (by now including a couple of stray lines) together:

Darien exclaimed, with no prompting from me, “It’s a half-pizza!” I was surprised, and mathematically pleased, with this description because using transformations to prove the Triangle Angle Sum Theorem involves performing half-turns and exploiting the properties of half-turns. We ended the activity there, as neither of the kids had yet used a protractor.

After this enjoyable foray, I turned my attention to thinking about how to use Web Sketchpad (WSP) to support Sarah’s lesson, and I came up with the two websketches below. The first can be shown to the full class in advance of the experiment itself, to give students a clear idea of what they will do, and how they will transition from the vague idea of turning their gaze (that is, their eyes) through an angle to the more specific process of each of the three team members, in her role as a vertex, accurately recording on paper the angle from one of her partners to the other. This first websketch below (and here) also introduces a link between the virtual world (the diagram and action in the websketch) and the physical world (in which students actually create a triangle and record its angles), thus preparing them to reverse the link (from physical to virtual) later in the lesson.

The second websketch below (and here) should be used, following the concrete physical activities of Sarah’s lesson, by small teams of students. It’s probably best to have teams of 3. with each team member doing a single vertex. Students use this sketch to construct a triangle, change its shape so that different teams are using different shapes, record the angle at each vertex, gather the three vertices of the angles, and rotate the angles so that all three are adjacent.

The first short hint video shows how to use the Record Angle tool by tapping in turn on three points that define the angle to be recorded: the initial direction of the turn, the vertex, and the final direction of the turn. The second hint video shows all three angles gathered and one of them being rotated to make it adjacent to another.

This second websketch is more meaningful to students after they’ve done the physical activity, particularly because the Record Angle tool produces a shape to represent an angle that’s similar to the shapes that students cut from paper to match their physical triangles. As Lakoff and Nuñez argue in Where Mathematics Come From, conceptual metaphors such as this cut-paper representation of an angle support the process through which students develop their abstract concept of “angle.” Similarly, the physical act of putting the three slices side-by-side around a common point supports students’ abstract concept of “adding angles.” This strategy—performing actions first with concrete physical objects (including their own bodies) and then with virtual objects using WSP— is a form of concreteness fading, an important technique for developing students’ abstract conceptual understanding.

This sketch has three blank pages so students can do the same experiment with three differently-shaped triangles and record their results by downloading their sketch. If students send their downloaded sketches to the teacher, she can use the Websketch Viewer to display all the sketches and choose the work of particular teams to give students a chance to describe their work and discuss with classmates.