Today’s guest post is from Marta Venturini, a PhD student in Mathematics Education at Simon Fraser University under a “Cotutelle Agreement” with the University of Bologna, where she’s a PhD student in Mathematics.

While looking for some tasks that would be suitable for Sketchpad,  I found the “dog leash” problem in a March 2007 Kangourou des Mathématiques test (The Kangourou Sans Frontières Association organizes the Kangourou game competition for more than four million participants from all over the world.) Here is the problem statement:

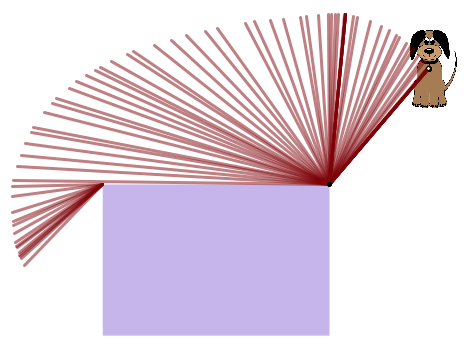
A leash with a maximum extension of 10 meters has one end attached to the corner of a rectangular house whose dimensions are 6 meters x 4 meters. A dog has his collar attached to the other end of the leash. The leash restricts how far the dog can wander around the lawn of the house. What is the area of the lawn where the dog can go without breaking the  leash?

I was drawn to this task because Sketchpad  acts as a visual amplifier for students as they explore the situation by dragging a dog around the screen. Sketchpad allows students to see where the dog can go within the constraints set by the leash and offers visual cues into the problem’s solution that would be difficult to obtain through paper-and-pencil exploration alone.

Download the sketch Dog Leash Problem.gsp and open it with Sketchpad (or you can view an online Web Sketchpad version here). Drag the dog around the screen and notice the limits of where it can go without the leash breaking.

To gain a better sense of the bounds imposed by the leash, press the Trace the Leash button. Now when you drag the dog, you’ll see the leash leave behind a trace of all its locations. Trace slowly and carefully to see the entire “accessible area” where the dog can roam.

Press the buttons circle1, circle2, and circle3 to view three circles whose location and size you can change simply by dragging them. Can you position the circles so that they represent the bounds imposed by the leash? Thinking about the radii of the circles and their placement may help you to determine the area in question.

The measure of the edges of the house can be set by the teacher, or students can explore the problem, changing the values of the parameters edge1 and edge2 (Note that the length of the leash is always equal to edge1+edge2.)

I’ve described one pathway through the problem, but your students will likely have their own insights to share. Let me know what they discover!