For the past eight months, my colleague Scott Steketee and I have collaborated with the authors of the elementary curriculum Everyday Mathematics to design interactive Web Sketchpad models for their next edition.
When it came time to create a Sketchpad representation of an isosceles triangle, I built the interactive triangle model below. Try dragging any of the triangle’s three vertices. You’ll see that while the angle measurements of ΔABC change, angles A and B always remain equal.
This isosceles triangle model is a a classic—a wonderful example of dynamic geometry’s ability to provide instantaneous feedback about a geometric construction as its parts are being dragged. As such, I expected the Everyday Mathematics authors to green-light the isosceles triangle without any discussion.
That didn’t happen.
The authors maintained that the model was too passive for young learners. Specifically, Sketchpad attended to the angle measurements, leaving the students to take on faith that the displayed measurements were correct. If students could measure the angles themselves, they not only would receive much-needed measurement practice, but they also might feel more invested in the discovery that angles A and B were always equal.
Based on this feedback, my initial instinct was to remove the angle measurements and include a virtual protractor that could be moved from vertex to vertex to measure the three angles. But this approach felt too cumbersome. I wanted students to measure the angles of as many isosceles triangles as possible, and if they only had one protractor, this process could become wearying.
My solution was to create the rather funny-looking model below with three mini protractors attached to ΔABC, one per vertex.
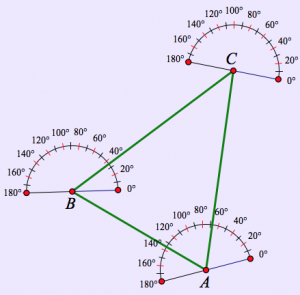
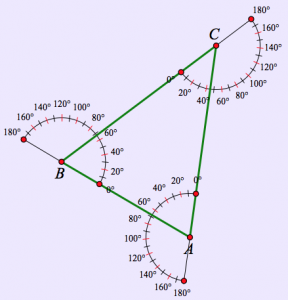
With this model, students can drag vertices A, B, and C to create a new triangle and then swing each of the three protractors into position to estimate the three angle measurements. The picture below shows the result of positioning all three protractors.
You can try this out for yourself and with your students by going to the second page of the interactive Web Sketchpad model.
The isosceles triangle is just one example of a sketch where my long-held opinions about Dynamic Geometry pedagogy were challenged by the Everyday Mathematics author team. I’m enjoying the opportunity to revisit and refine my beliefs!
Maybe slightly off topic, but are you able to say what grade level this activity is intended for? I’ve been wondering how closely EM will align with the Common Core and your sketch makes me even more curious…
Thanks for writing, Aaron. Everyday Mathematics is making extensive changes to their curriculum to align themselves with the Common Core. Our use of Web Sketchpad in EM fits particularly well with the Standards for Mathematical Practice, as Sketchpad explorations invariably lead to the development of key mathematical habits of mind. In the case of this triangle activity, no final decisions have been made yet, but I expect it may be used in grade 4.
Thanks for sharing these two different sketches Daniel. I learned something valuable, from this subtle, but I think significant, shift from the first to the second sketch, in how we can ask students to be a little more active in their learning. I agree with the EM authors that students might well learn more from their interactions with the second sketch, as long as they have previous experience with interpreting protractor measures. Otherwise they might get tripped up on that here, and not be able to focus on the key learning about isosceles triangles.
Thanks for the comments, Rick. We’re definitely planning to provide introductory protractor activities in which students will use a virtual protractor to measure a single angle. With these activities as a foundation, I think it’s likely that students will feel confident in their use of a protractor by the time they reach this isosceles triangle activity.