[This guest post by Sarah Stephens, a senior at Pennsylvania State University, describes a lesson she created as part of her Senior Honors Thesis on leveraging embodied cognition to help students develop abstract mathematical concepts.]

As a soon-to-be classroom mathematics teacher, I have taken special interest in the field of embodied cognition and integrating it into the mathematics classroom. I have been working to develop lessons that incorporate purposeful movement (movement directly related to the mathematics content) so that students can embody mathematical ideas. Incorporating movement in this way is a type of problem-based learning that emphasizes student-centered lessons; however, the goal for these lessons is to go one step further than problem-based lessons by grounding students’ learning in their physical experiences, directly linking students to the mathematics.

When choosing mathematical content to embody, I first focus on a mathematical concept and then figure out how it can be related to a students’ physical actions. It is important that the physical action is simple and natural, something that occurs in our everyday experiences. Such a movement can become a conceptual metaphor for students—a metaphor that helps them to better understand an abstract mathematical concept by linking it to their bodily action. Everyday movements that I have explored with students include walking, dancing, and turning (rotating).

In the lesson described below, I started by thinking about the abstract idea of angle, and then brainstormed what bodily movement would best represent it. Often in elementary school, and even sometimes middle school, teachers describe angles through the concept of turning. I decided to extend this idea by creating a lesson that asked students to explore angles and, in particular, angle sums, by grounding it in their physical experience of turning and rotating. The collection of two lessons explores finding the exterior angle sum of polygons in the first lesson, and the interior angle sum of polygons in the second. In the interest of length, this blog will just discuss finding the interior angle sum of a triangle.

Exploring The Interior Angle Sum of a Triangle

Most high-school graduates have been exposed to the formula S(n) = 180° (n – 2) and could recognize it as the general formula to find the interior angle sum of a polygon with n sides. The majority of high school students that I have encountered have been expected to memorize this equation at some point in their geometry career. While some students may be able to explain why this equation works, most cannot. Learning this formula is often a boring lesson focused on memorization and simply plugging in numbers to find an answer. My goal was to create a lesson using embodiment to reinforce conceptual understanding of what an angle is, what it means to measure an angle, and what it means to find the sum of angles.

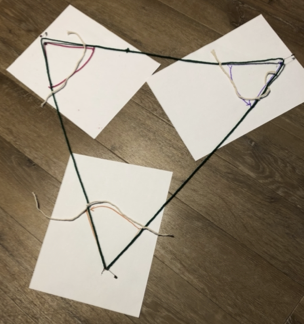
Embodiment came through having one student represent each vertex of a triangle so that a team of three students represented the skeleton of the shape. Each student’s location represented the fixed point of rotation for the angle they would act out. Each team used a closed loop of string, held and pulled taught by the student “vertices,” to form the perimeter of the triangle. Before having students embody the angle, it was important to clarify what an angle was—to make sure they knew what they were embodying. The idea of an angle used for this lesson was “the space between two rays that share a common endpoint.” However, in this lesson, students understood that angles were not static, and that the angle itself was represented by a turn through space: the change in position from where one starts and where one ends after turning. 

In this activity, students began by practicing the movement. Each student stood at their vertex facing the classmate at the vertex to their right, with one arm straight out pointing to the adjacent vertex. Then they turned counter clockwise through the angle until they were now facing the vertex (student) to their left. Going through this motion fully embodied the angle.

Before moving on, the lesson asks each student to repeat this process, this time holding a pre-cut second piece of string so that its loose end drags on the floor, leaving an arc that records their arm movement. This pre-cut string concretely shows them what their embodiment is measuring and reinforces the idea that the angle is a measure of the rotation of their arm.

Finally, students notate their movement on a sheet of paper to record the measurement of the angle they just embodied. They put a piece of paper on the floor, mark an actual point as their vertex, and draw an arrow pointing to each of the adjacent vertices. The end of the loose string is then represented as a directed arc on their paper, as shown in the picture below.

The embodiment continues with students cutting each paper diagram to remove the portions of the paper external to the polygon, so that the remaining wedge is a physical (paper) semi-abstract representation of the student’s physical turn.

Once each student has cut their measurement, the three papers can be put together in a way that shows the sum of the angles is a straight or 180° angle. The cut paper now represents both the individual angle measurements of students as well as the sum of the angles in a more physical and less abstract way. The image below illustrates the use of paper to measure the angle sum concretely without resorting to protractors and numeric measurements.

At this point, students can use protractors to confirm that the resulting sum of the angles is approximately 180° by measuring the angle of their combined paper representation. Using the paper to embody the angles provides students with a representation of the angle sum that is more concrete and memorable than a numeric sum of protractor measurements.

Another benefit of this lesson is that multiple groups will do it at the same time, with each group creating a different triangle triangle. When comparing their paper cut outs, all groups will notice that despite having different triangles, their sums all create a straight angle, meaning that for each group, no matter the measure of the individual angles, the total rotation will always be 180°. 

This has the potential of being a powerful lesson. One of the reasons why purposeful movement effectively helps students understand mathematical concepts is because it allows them to process material concretely before moving to abstraction. Helping students understand abstract concepts has been something that I have struggled with as a classroom teacher. However, my students have had success in learning when I first introduce concrete representations and incorporate movement. Integrating purposeful movement into a mathematics classroom first allows students to understand the mathematics concretely (through their bodies) and then gradually understand abstract ideas as they begin to make connections between their actions and mathematical ideas.

[The process Sarah describes, of using students’ concrete actions as the grounds on which to build their abstract understanding of a mathematical concept, can be further supported by dynamic math software. A follow-up post, coming soon, will provide a Web Sketchpad model to support Sarah’s lesson,]