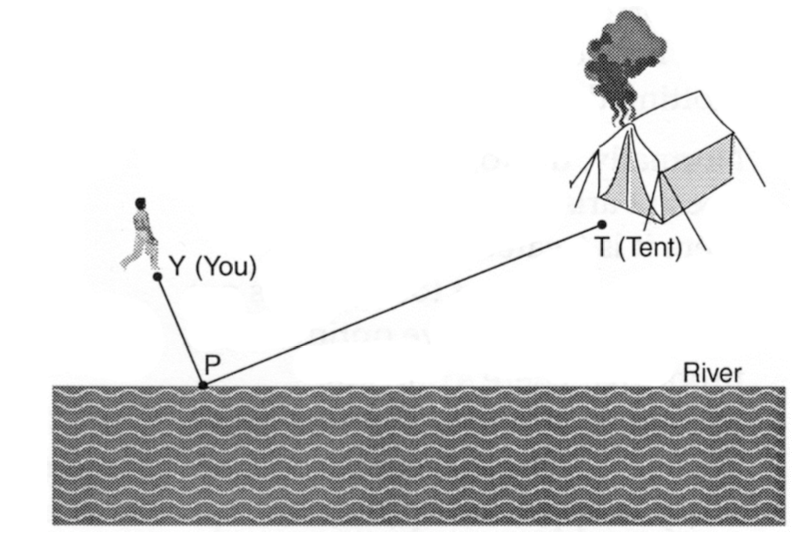
How can you identify a lover of math? Casually mention a burning tent and notice if her first thought is how to minimize her path to a river and then to the tent to douse the flames. Here is a full statement of this classic geometry problem:

Ah, the great outdoors. Camping, the fresh air, the starry night, and—fire! Your tent is ablaze! Fortunately, you (at point Y) have a bucket in hand. You decide to run to the river’s edge, fill your bucket, and race to your tent (point T) to extinguish the fire. Where along the river should you head in order to minimize your total running distance? The picture below shows one possible location—point P—where you might run.

The standard approach to solving this problem requires a flash of insight: If point T is reflected across the river’s edge to create a point T’, then running from Y to P to T is the same distance as running from running from Y to P to T’. So to find the best location for P, simply connect points Y and T’ and mark where the segment intersects the river’s edge.

You can explore this solution approach on page 1 of the Web Sketchpad model that appears at the end of this post. The video below it provides an overview of how to use Web Sketchpad’s construction tools.

Usually, the Burning Tent problem ends here. But I’d like to propose an entirely different approach to solving the problem, one that comes from EDC‘s Connected Geometry curriculum.

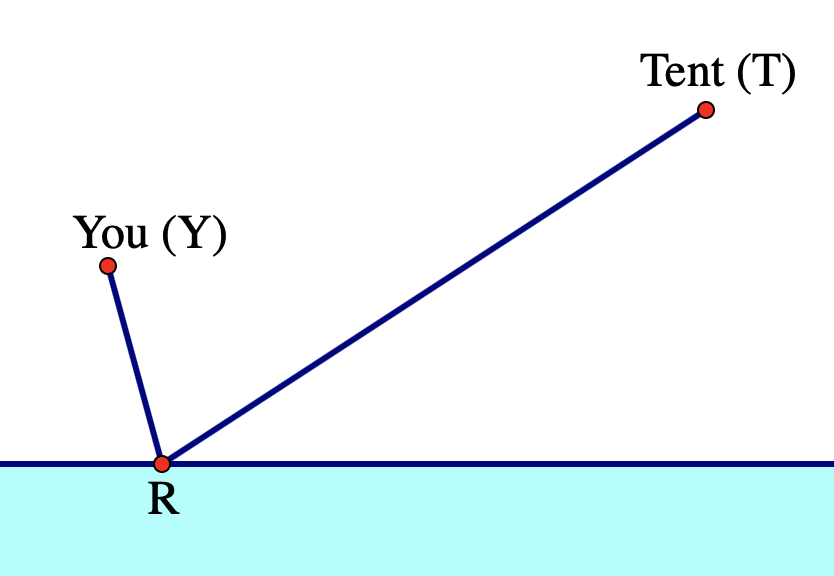
Suppose that instead of searching for the best spot along the river, you just pick any spot, like point R below.

What is the set of all points on the plane that are no better or worse than point R? In other words, find all points P such that YR + RT = YP + PT. For those of you who are familiar with the distance definition of an ellipse, you’ll recognize that these locations can be found by creating an ellipse with foci at points Y and T that passes through point R (see the picture below).

How can this ellipse help us to to identify better spots along the river? And how, by adjusting the location of point R and its accompanying ellipse, can we find the best spot?

Drawing one ellipse, let alone multiple ellipses, is a lot of work if you’re doing so by hand, but Web Sketchpad makes it super easy to create an ellipse that adjusts dynamically as you drag point R. The video at the end of this post explains how you can do so by using page 2 of the Web Sketchpad model.

You can read much more about this approach to solving optimization problems like the Burning Tent in Connected Geometry’s optimization chapter. In my next post, I’ll apply the ellipse approach to solving the Cowgirl problem—a fun extension of the burning tent.