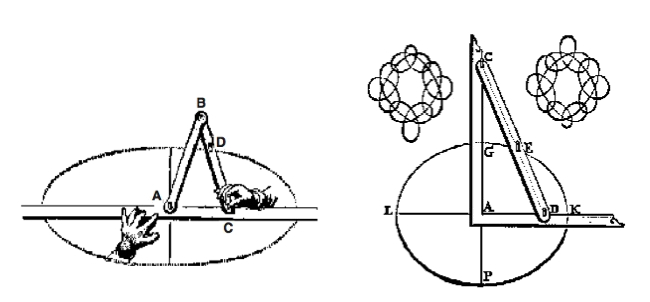
The 17th-century Dutch mathematician Frans van Schooten developed “hands-on manipulatives” centuries before the term became popular in math education circles. Below are two images of ellipse-drawing linkages from van Schooten’s manuscript, Sive de Organica Conicarum Sectionum in Plano Descriptione, Tractatus (A Treatise on Devices for Drawing Conic Sections).

Building physical models of these devices isn’t hard. A bent straw, for example, works well for the linkage on the left.  As another approach, you can use Sketchpad to construct them.

The interactive Web Sketchpad models below allow you to draw ellipses using the two models above as well as two other related methods. For each model, press the Animate button to set it in motion. You can move between the models using the arrows in the bottom-right corner of the sketch window.

For each model, experiment with different locations of the green point that’s tracing the ellipse. How does the shape of the ellipse change based on the point’s position? You can also experiment with the length controls at the bottom left of each page.

It’s not especially difficult to prove that these four models do, in fact, draw ellipses. The Show Proof Hints button on the Bent Straw page offers some ideas that you can apply to the other two models as well.

If you’d like to explore other methods of constructing ellipses, check out my prior blog posts, Danny’s Ellipse, The Congruent Triangle Construction, and The Tangent Circles Construction. You’ll find these and many more conic section constructions in my book, Exploring Conic Sections with The Geometer’s Sketchpad.